Syllabus for Master of Science (Mathematics)
Course Details
Eligibility Criteria
Bachelor of Science (B.Sc) in Mathematics
Intake
30 Seats
Duration
The duration of the course shall be Full time two academic years. Each academic year shall be divided into two semesters.
Curriculum
SEMESTER - 1
 Teaching Evaluation Scheme
Teaching Evaluation Scheme
| Subjects |
|---|
| 040410101 Topology |
| 040410102 Functional Analysis |
| 040410103 Advanced Numerical Analysis |
| 040410104 Advanced Partial Differential Equations |
| 040410105 Fuzzy Logic |
| 040410106 Statistical Decision Theory |
| 040410107 Mathematics of Financial Derivatives |
SEMESTER - 2
 Teaching Evaluation Scheme
Teaching Evaluation Scheme
SEMESTER - 3
 Teaching Evaluation Scheme
Teaching Evaluation Scheme
SEMESTER - 4
 Teaching Evaluation Scheme
Teaching Evaluation Scheme
Fee Structure
1. Tuition Fee
25,000
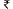 Per Semester
Per Semester
2. Other Fee
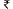 3,250 Per Semester
3,250 Per Semester
(Including Student Welfare, Books, Equipment, Campus Development, Internal Examination,Sports and Cultural Activities)
3. One Time Fee
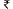 6,150 At time of admission
6,150 At time of admission
(It includes College Deposit(Refundable),Alumni MemberShip fees,Registration, and I-Card)
Programme Outcome (PO) & Programme Specific Outcomes (PSO)
Programme Outcome (PO)
- PO 1: Knowledge: Provides knowledge about the fundamentals of pure, applied and computing mathematics and its applications to students that creates the opportunitiesin industries and research centers.
- PO 2: Core Competence: Creates competency in science and mathematics to formulate, analyses and solve problem and/or also to pursue advanced study or research.
- PO 3:Breadth: Trainsstudents having good knowledge in unearth core of academia and industry by the roots of mathematics.
- PO 4: Evaluation: Imparts in students to raise trial and error based curiosity and problem solving functionality with research based advanced tutorial for higher level decision makings tools.
Programme Specific Outcomes (PSO)
- PSO-1:Understand thefundamental principles underlying the major areas of mathematics.
- PSO-2: Evaluatealogical, reasoning andmathematics savvy approach.
- PSO-3: Explorea newer way of mathematical applications.
- PSO-4: Create an attribute towards problem solving and research orientation.
- PSO-5: Able to apply analytical and theoretical skills to model and solve mathematical problems.
- PSO-6: Develop mathematical computing skills to solve complex mathematical models.